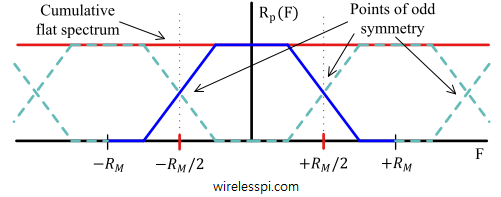
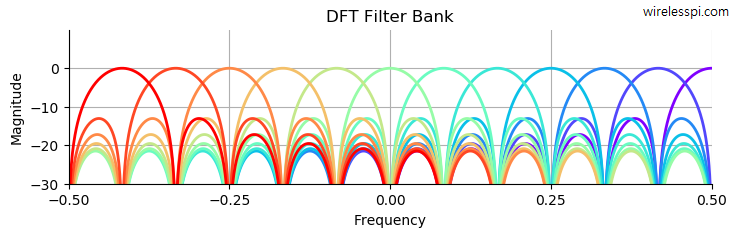
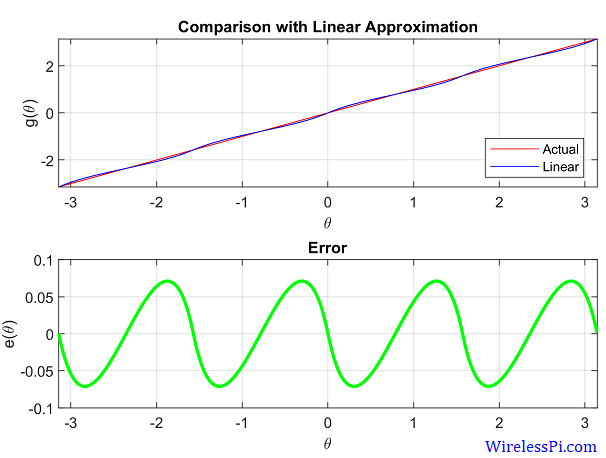
June 18, 2020 On a cold morning in August 2015, I narrowly missed a train to my office in Melbourne city. With nothing else to do in the next 20 minutes, my mind wandered towards an intuitive view of complex numbers, something that has puzzled me since long. In particular, I wanted to seek answers to the following questions. (a) What is the role of the number $\sqrt{-1}$ in mathematics? What sets it apart from other impossible numbers, e.g., a number $k$ such that $|k|=-1$? (The origins of this question might lie in how I cut apple slices for my
Continue reading